How To Draw The Molecular Orbital Diagram

Chapter 8. Advanced Theories of Covalent Bonding

viii.4 Molecular Orbital Theory

Learning Objectives

By the end of this section, you will be able to:

• Outline the bones quantum-mechanical approach to deriving molecular orbitals from diminutive orbitals
• Describe traits of bonding and antibonding molecular orbitals
• Summate bond orders based on molecular electron configurations
• Write molecular electron configurations for first- and second-row diatomic molecules
• Relate these electron configurations to the molecules' stabilities and magnetic backdrop

For nigh every covalent molecule that exists, nosotros tin can now depict the Lewis structure, predict the electron-pair geometry, predict the molecular geometry, and come up shut to predicting bond angles. Nevertheless, one of the well-nigh of import molecules we know, the oxygen molecule O_ii, presents a trouble with respect to its Lewis structure. Nosotros would write the following Lewis structure for O_two:

This electronic construction adheres to all the rules governing Lewis theory. There is an O=O double bond, and each oxygen atom has eight electrons around it. Withal, this film is at odds with the magnetic beliefs of oxygen. By itself, O_ii is not magnetic, but it is attracted to magnetic fields. Thus, when we pour liquid oxygen by a strong magnet, it collects between the poles of the magnet and defies gravity, equally in Figure 1 in Chapter viii Introduction. Such attraction to a magnetic field is called paramagnetism, and it arises in molecules that take unpaired electrons. And yet, the Lewis structure of O₂ indicates that all electrons are paired. How practise we business relationship for this discrepancy?

Magnetic susceptibility measures the force experienced past a substance in a magnetic field. When nosotros compare the weight of a sample to the weight measured in a magnetic field (Figure 1), paramagnetic samples that are attracted to the magnet will appear heavier because of the force exerted by the magnetic field. We can summate the number of unpaired electrons based on the increase in weight.

Effigy ane. A Gouy balance compares the mass of a sample in the presence of a magnetic field with the mass with the electromagnet turned off to determine the number of unpaired electrons in a sample.

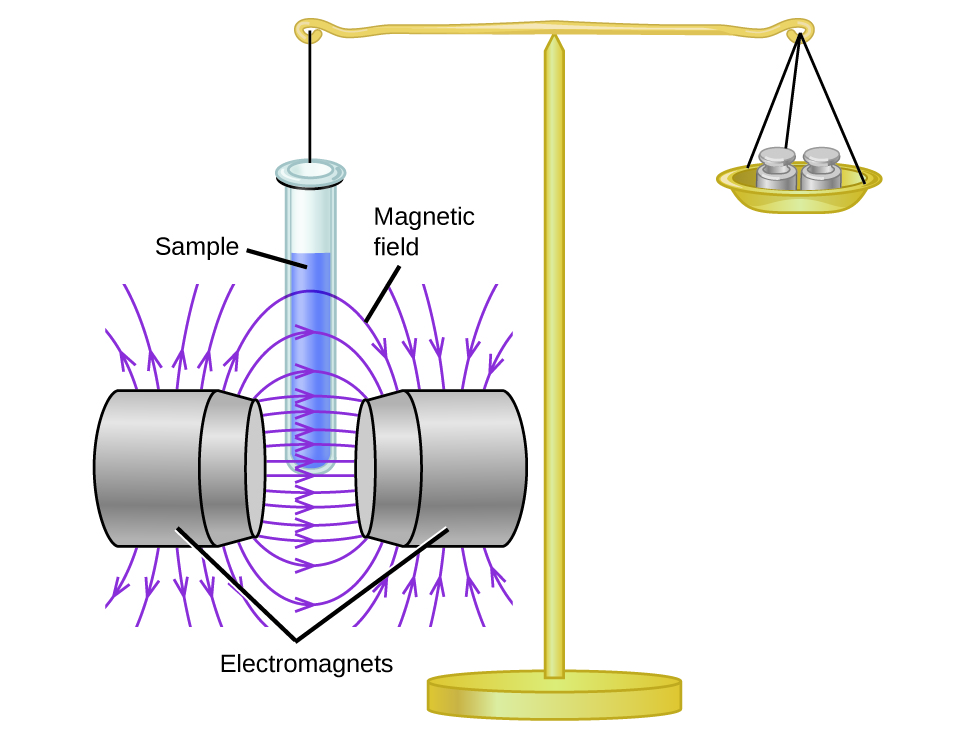
Experiments prove that each O_two molecule has two unpaired electrons. The Lewis-structure model does non predict the presence of these two unpaired electrons. Unlike oxygen, the apparent weight of near molecules decreases slightly in the presence of an inhomogeneous magnetic field. Materials in which all of the electrons are paired are diamagnetic and weakly repel a magnetic field. Paramagnetic and diamagnetic materials do not act as permanent magnets. Only in the presence of an applied magnetic field do they demonstrate attraction or repulsion.

H2o, like near molecules, contains all paired electrons. Living things contain a large percentage of water, and then they demonstrate diamagnetic behavior. If you lot place a frog near a sufficiently big magnet, it volition levitate. You can see videos of diamagnetic floating frogs, strawberries, and more than.

Molecular orbital theory (MO theory) provides an explanation of chemic bonding that accounts for the paramagnetism of the oxygen molecule. Information technology also explains the bonding in a number of other molecules, such as violations of the octet dominion and more than molecules with more complicated bonding (beyond the telescopic of this text) that are hard to describe with Lewis structures. Additionally, it provides a model for describing the energies of electrons in a molecule and the probable location of these electrons. Different valence bail theory, which uses hybrid orbitals that are assigned to one specific atom, MO theory uses the combination of diminutive orbitals to yield molecular orbitals that are delocalized over the unabridged molecule rather than beingness localized on its constituent atoms. MO theory also helps us understand why some substances are electric conductors, others are semiconductors, and all the same others are insulators. Table ii summarizes the master points of the two complementary bonding theories. Both theories provide different, useful means of describing molecular structure.

Valence Bond Theory	Molecular Orbital Theory
considers bonds as localized between one pair of atoms	considers electrons delocalized throughout the entire molecule
creates bonds from overlap of atomic orbitals (south, p, d…) and hybrid orbitals (sp, sp two, sp three…)	combines atomic orbitals to form molecular orbitals (σ, σ*, π, π*)
forms σ or π bonds	creates bonding and antibonding interactions based on which orbitals are filled
predicts molecular shape based on the number of regions of electron density	predicts the arrangement of electrons in molecules
needs multiple structures to draw resonance
Tabular array 2. Comparison of Bonding Theories

Molecular orbital theory describes the distribution of electrons in molecules in much the same mode that the distribution of electrons in atoms is described using atomic orbitals. Using quantum mechanics, the beliefs of an electron in a molecule is still described by a moving ridge function, Ψ, analogous to the behavior in an atom. Just like electrons around isolated atoms, electrons effectually atoms in molecules are limited to discrete (quantized) energies. The region of space in which a valence electron in a molecule is probable to be establish is called a molecular orbital (Ψ ²). Like an diminutive orbital, a molecular orbital is full when it contains two electrons with contrary spin.

Nosotros volition consider the molecular orbitals in molecules composed of two identical atoms (H_ii or Cl_two, for example). Such molecules are called homonuclear diatomic molecules. In these diatomic molecules, several types of molecular orbitals occur.

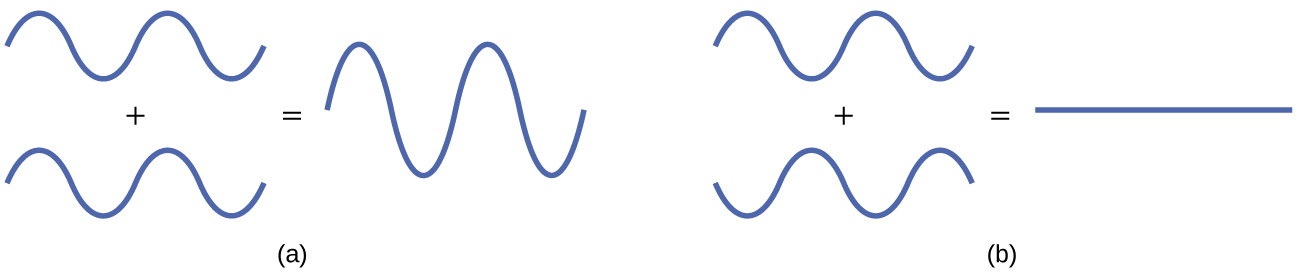
The mathematical process of combining atomic orbitals to generate molecular orbitals is called the linear combination of atomic orbitals (LCAO). The wave function describes the wavelike properties of an electron. Molecular orbitals are combinations of diminutive orbital moving ridge functions. Combining waves tin lead to constructive interference, in which peaks line up with peaks, or destructive interference, in which peaks line up with troughs (Effigy 2). In orbitals, the waves are three dimensional, and they combine with in-phase waves producing regions with a higher probability of electron density and out-of-phase waves producing nodes, or regions of no electron density.

Figure 2. (a) When in-stage waves combine, constructive interference produces a wave with greater amplitude. (b) When out-of-phase waves combine, destructive interference produces a moving ridge with less (or no) amplitude.

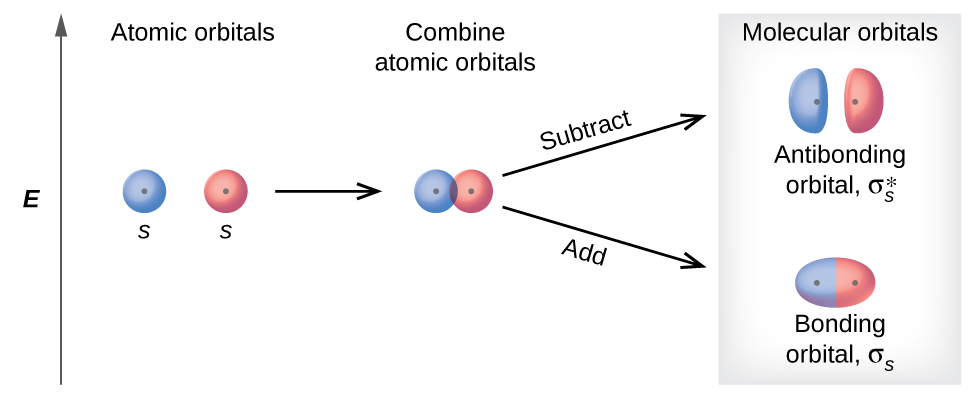
There are ii types of molecular orbitals that can class from the overlap of two atomic s orbitals on side by side atoms. The two types are illustrated in Figure 3. The in-stage combination produces a lower energy σ _s molecular orbital (read as "sigma-south") in which almost of the electron density is directly betwixt the nuclei. The out-of-phase addition (which can also be thought of as subtracting the wave functions) produces a higher energy [latex]\pmb \sigma^*_s[/latex]molecular orbital (read as "sigma-s-star") molecular orbital in which there is a node between the nuclei. The asterisk signifies that the orbital is an antibonding orbital. Electrons in a σ _s orbital are attracted past both nuclei at the same time and are more than stable (of lower energy) than they would be in the isolated atoms. Adding electrons to these orbitals creates a forcefulness that holds the ii nuclei together, so we telephone call these orbitals bonding orbitals. Electrons in the [latex]\sigma^*_s[/latex] orbitals are located well away from the region between the two nuclei. The attractive force betwixt the nuclei and these electrons pulls the two nuclei apart. Hence, these orbitals are called antibonding orbitals. Electrons fill the lower-energy bonding orbital before the higher-energy antibonding orbital, just as they fill lower-energy diminutive orbitals before they make full higher-energy atomic orbitals.

Figure three. Sigma (σ) and sigma-star (σ*) molecular orbitals are formed by the combination of two s atomic orbitals. The plus (+) signs indicate the locations of nuclei.

You can spotter animations visualizing the calculated diminutive orbitals combining to class various molecular orbitals at the Orbitron website.

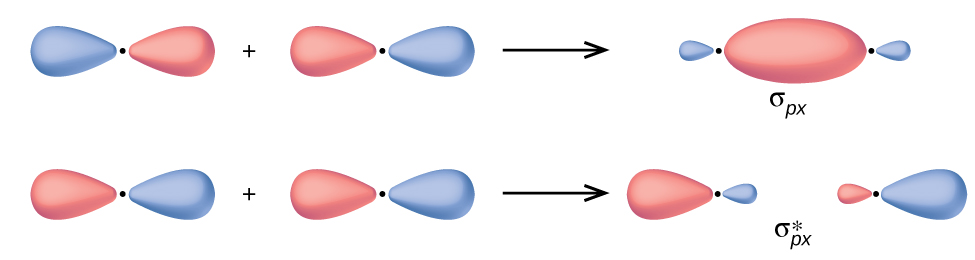
In p orbitals, the moving ridge office gives rising to 2 lobes with opposite phases, analogous to how a 2-dimensional moving ridge has both parts above and below the average. We indicate the phases by shading the orbital lobes unlike colors. When orbital lobes of the same phase overlap, constructive wave interference increases the electron density. When regions of opposite phase overlap, the subversive wave interference decreases electron density and creates nodes. When p orbitals overlap end to terminate, they create σ and σ* orbitals (Figure four). If two atoms are located forth the x-axis in a Cartesian coordinate organization, the 2 p_ten orbitals overlap stop to stop and class σ _px (bonding) and [latex]\sigma^*_{px}[/latex] (antibonding) (read as "sigma-p-x" and "sigma-p-ten star," respectively). Just as with s-orbital overlap, the asterisk indicates the orbital with a node betwixt the nuclei, which is a higher-energy, antibonding orbital.

Figure four. Combining moving ridge functions of two p atomic orbitals forth the internuclear axis creates two molecular orbitals, σ _p and σ* _p .

The side-past-side overlap of ii p orbitals gives ascension to a pi (π) bonding molecular orbital and a π* antibonding molecular orbital, equally shown in Figure v. In valence bond theory, we draw π bonds as containing a nodal airplane containing the internuclear centrality and perpendicular to the lobes of the p orbitals, with electron density on either side of the node. In molecular orbital theory, we draw the π orbital by this same shape, and a π bond exists when this orbital contains electrons. Electrons in this orbital collaborate with both nuclei and aid hold the two atoms together, making it a bonding orbital. For the out-of-phase combination, there are two nodal planes created, ane along the internuclear axis and a perpendicular one between the nuclei.

Figure 5. Side-past-side overlap of each two p orbitals results in the formation of two π molecular orbitals. Combining the out-of-phase orbitals results in an antibonding molecular orbital with ii nodes. One contains the internuclear axis, and ane is perpendicular to the axis. Combining the in-phase orbitals results in a bonding orbital. There is a node (blue) containing the internuclear axis with the ii lobes of the orbital located in a higher place and below this node.

In the molecular orbitals of diatomic molecules, each atom also has two sets of p orbitals oriented side past side (p_y and p_z ), so these 4 diminutive orbitals combine pairwise to create two π orbitals and two π* orbitals. The π _py and [latex]\pi^*_{py}[/latex] orbitals are oriented at right angles to the π _pz and [latex]\pi^*_{pz}[/latex] orbitals. Except for their orientation, the π _py and π _pz orbitals are identical and take the same free energy; they are degenerate orbitals. The [latex]\pi^*_{py}[/latex] and [latex]\pi^*_{px}[/latex] antibonding orbitals are likewise degenerate and identical except for their orientation. A total of half-dozen molecular orbitals results from the combination of the vi atomic p orbitals in 2 atoms: σ _px and [latex]\sigma^*_{px}[/latex], π _py and [latex]\pi^*_{py}[/latex], π _pz and [latex]\pi^*_{pz}[/latex].

Example 1

Molecular Orbitals
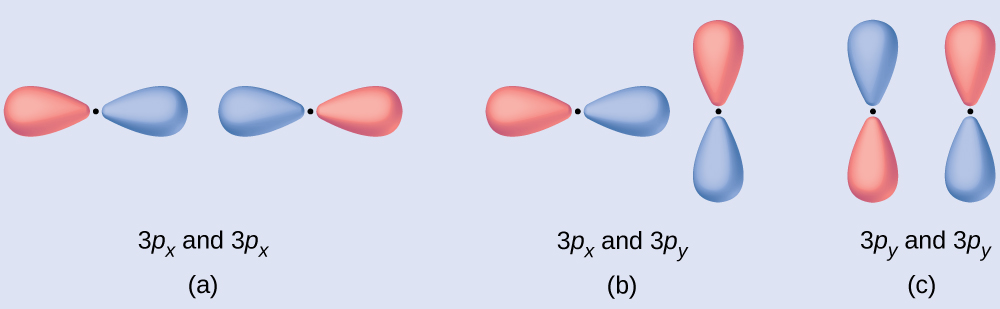
Predict what type (if whatsoever) of molecular orbital would effect from adding the wave functions and so each pair of orbitals shown overlap. The orbitals are all similar in energy.

Solution
(a) is an in-phase combination, resulting in a σ_3p orbital

(b) will not result in a new orbital because the in-stage component (lesser) and out-of-stage component (top) cancel out. Only orbitals with the right alignment tin can combine.

(c) is an out-of-stage combination, resulting in a [latex]\pi^*_{3p}[/latex] orbital.

Check Your Learning
Label the molecular orbital shown as σ or π, bonding or antibonding and point where the node occurs.

Reply:

The orbital is located along the internuclear axis, so it is a σ orbital. There is a node bisecting the internuclear axis, so it is an antibonding orbital.

Walter Kohn: Nobel Laureate

Walter Kohn (Figure six) is a theoretical physicist who studies the electronic structure of solids. His work combines the principles of quantum mechanics with avant-garde mathematical techniques. This technique, called density functional theory, makes it possible to compute properties of molecular orbitals, including their shape and energies. Kohn and mathematician John Pople were awarded the Nobel Prize in Chemistry in 1998 for their contributions to our understanding of electronic construction. Kohn likewise fabricated pregnant contributions to the physics of semiconductors.

Effigy six. Walter Kohn developed methods to draw molecular orbitals. (credit: image courtesy of Walter Kohn)

Kohn'southward biography has been remarkable outside the realm of physical chemistry as well. He was born in Republic of austria, and during World War II he was role of the Kindertransport programme that rescued 10,000 children from the Nazi authorities. His summertime jobs included discovering gold deposits in Canada and helping Polaroid explain how its instant film worked. Although he is now an emeritus professor, he is still actively working on projects involving global warming and renewable free energy.

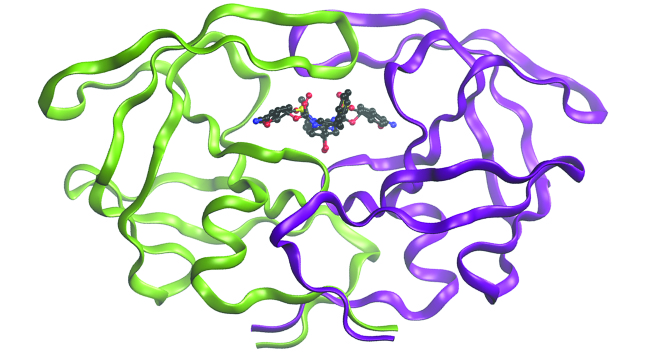
Computational Chemistry in Drug Design

While the descriptions of bonding described in this chapter involve many theoretical concepts, they as well accept many practical, existent-world applications. For example, drug design is an of import field that uses our understanding of chemical bonding to develop pharmaceuticals. This interdisciplinary area of study uses biology (understanding diseases and how they operate) to place specific targets, such as a binding site that is involved in a disease pathway. By modeling the structures of the binding site and potential drugs, computational chemists can predict which structures tin fit together and how effectively they will bind (see Figure 7). Thousands of potential candidates can exist narrowed down to a few of the most promising candidates. These candidate molecules are then carefully tested to determine side effects, how effectively they tin can be transported through the body, and other factors. Dozens of important new pharmaceuticals have been discovered with the assistance of computational chemistry, and new research projects are underway.

Figure 7. The molecule shown, HIV-ane protease, is an of import target for pharmaceutical inquiry. By designing molecules that demark to this protein, scientists are able to drastically inhibit the progress of the affliction.

Molecular Orbital Free energy Diagrams

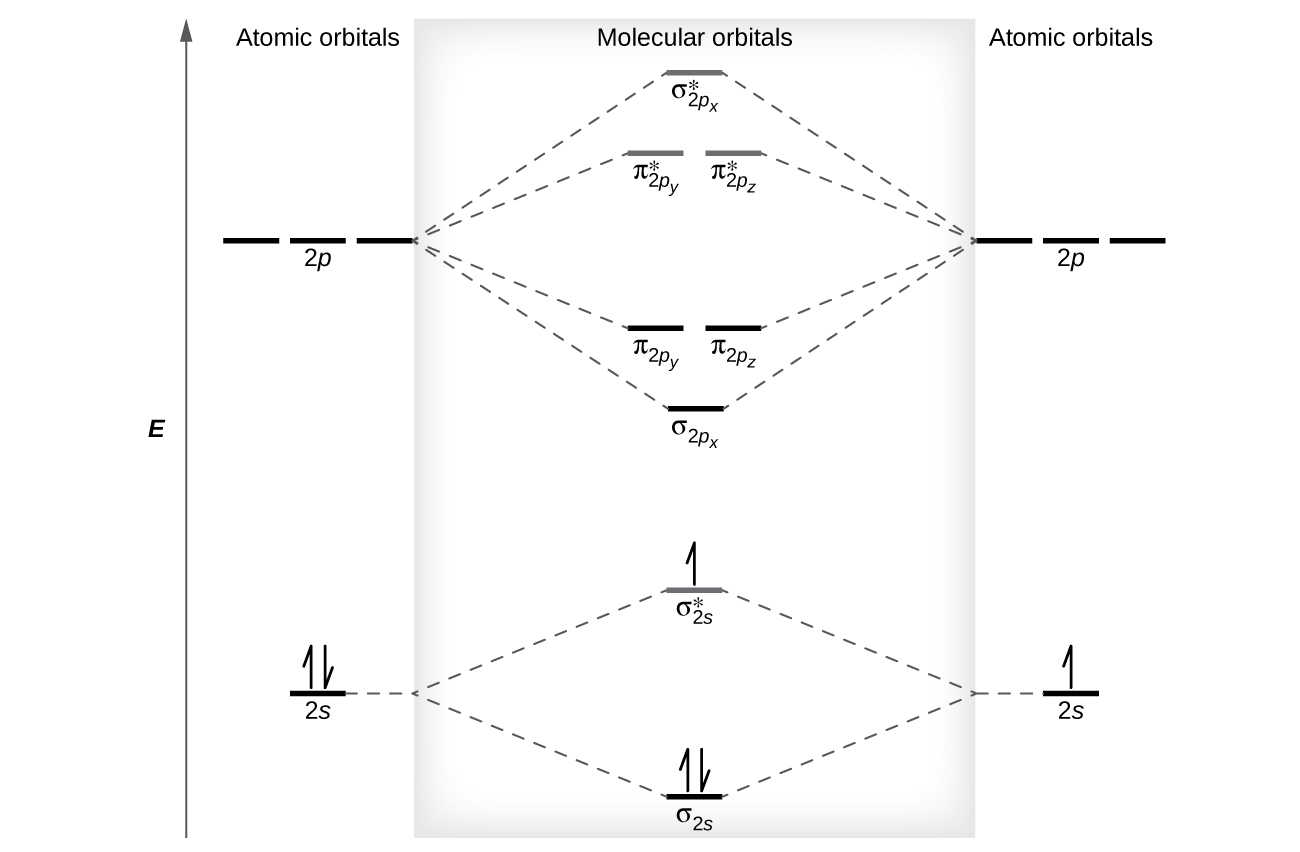
The relative energy levels of atomic and molecular orbitals are typically shown in a molecular orbital diagram (Figure 8). For a diatomic molecule, the diminutive orbitals of one atom are shown on the left, and those of the other atom are shown on the right. Each horizontal line represents ane orbital that tin hold 2 electrons. The molecular orbitals formed past the combination of the diminutive orbitals are shown in the middle. Dashed lines bear witness which of the atomic orbitals combine to form the molecular orbitals. For each pair of atomic orbitals that combine, one lower-free energy (bonding) molecular orbital and one higher-energy (antibonding) orbital result. Thus we tin can come across that combining the 6 2p atomic orbitals results in three bonding orbitals (one σ and two π) and three antibonding orbitals (one σ* and ii π*).

We predict the distribution of electrons in these molecular orbitals by filling the orbitals in the aforementioned mode that we fill atomic orbitals, by the Aufbau principle. Lower-energy orbitals fill showtime, electrons spread out among degenerate orbitals earlier pairing, and each orbital can agree a maximum of two electrons with opposite spins (Figure eight). Just every bit we write electron configurations for atoms, we can write the molecular electronic configuration past listing the orbitals with superscripts indicating the number of electrons nowadays. For clarity, nosotros place parentheses around molecular orbitals with the same energy. In this example, each orbital is at a dissimilar energy, then parentheses separate each orbital. Thus nosotros would expect a diatomic molecule or ion containing seven electrons (such every bit Be₂ ⁺) would have the molecular electron configuration [latex](\sigma_{1s})^ii[/latex] [latex](\sigma^*_{1s})^2[/latex] [latex](\sigma_{2s})^ii[/latex] [latex](\sigma^*_{2s})^1[/latex]. It is mutual to omit the cadre electrons from molecular orbital diagrams and configurations and include only the valence electrons.

Figure eight. This is the molecular orbital diagram for the homonuclear diatomic Be₂ ⁺, showing the molecular orbitals of the valence shell merely. The molecular orbitals are filled in the same manner as atomic orbitals, using the Aufbau principle and Hund's rule.

Bond Guild

The filled molecular orbital diagram shows the number of electrons in both bonding and antibonding molecular orbitals. The net contribution of the electrons to the bail force of a molecule is identified by determining the bond order that results from the filling of the molecular orbitals by electrons.

When using Lewis structures to depict the distribution of electrons in molecules, we define bond order as the number of bonding pairs of electrons betwixt ii atoms. Thus a single bond has a bail club of 1, a double bail has a bond order of 2, and a triple bond has a bond gild of 3. We define bond guild differently when we apply the molecular orbital description of the distribution of electrons, but the resulting bond gild is commonly the same. The MO technique is more accurate and can handle cases when the Lewis structure method fails, but both methods describe the same phenomenon.

In the molecular orbital model, an electron contributes to a bonding interaction if it occupies a bonding orbital and information technology contributes to an antibonding interaction if it occupies an antibonding orbital. The bond order is calculated past subtracting the destabilizing (antibonding) electrons from the stabilizing (bonding) electrons. Since a bail consists of two electrons, we divide past two to go the bail order. Nosotros tin determine bond order with the following equation:

[latex]\text{bond order} = \frac{(\text{number of bonding electrons}) - (\text{number of antibonding electrons})}{two}[/latex]

The order of a covalent bond is a guide to its strength; a bond between two given atoms becomes stronger equally the bail gild increases (Tabular array i in Chapter 8.1 Valence Bond Theory). If the distribution of electrons in the molecular orbitals between 2 atoms is such that the resulting bail would have a bond social club of zero, a stable bond does non grade. We next await at some specific examples of MO diagrams and bail orders.

Bonding in Diatomic Molecules

A dihydrogen molecule (H₂) forms from two hydrogen atoms. When the diminutive orbitals of the two atoms combine, the electrons occupy the molecular orbital of everyman energy, the σ_1south bonding orbital. A dihydrogen molecule, H₂, readily forms considering the free energy of a H₂ molecule is lower than that of two H atoms. The σ_1southward orbital that contains both electrons is lower in energy than either of the 2 1s diminutive orbitals.

A molecular orbital can agree 2 electrons, and so both electrons in the H_ii molecule are in the σ_anesouth bonding orbital; the electron configuration is [latex](\sigma_{1s})^ii[/latex]. Nosotros represent this configuration past a molecular orbital energy diagram (Figure 9) in which a single upward arrow indicates one electron in an orbital, and two (upward and downward) arrows signal two electrons of contrary spin.

Figure 9. The molecular orbital free energy diagram predicts that H₂ will exist a stable molecule with lower energy than the separated atoms.

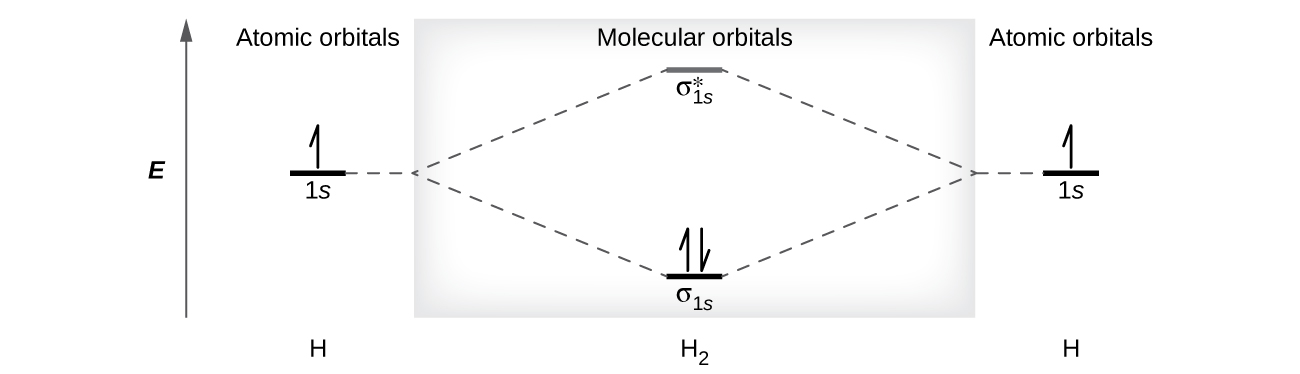
A dihydrogen molecule contains two bonding electrons and no antibonding electrons and then we have

[latex]\text{bail order in H}_2 = \frac{(2 - 0)}{2} = ane[/latex]

Because the bail order for the H–H bail is equal to 1, the bond is a single bail.

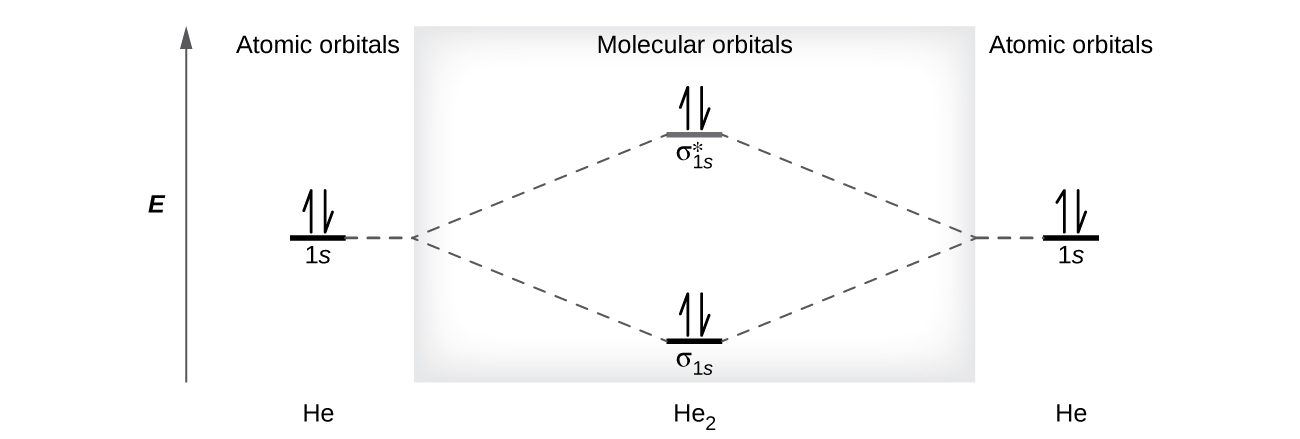
A helium atom has two electrons, both of which are in its 1s orbital. Two helium atoms do not combine to form a dihelium molecule, He₂, with four electrons, because the stabilizing effect of the two electrons in the lower-energy bonding orbital would be offset by the destabilizing effect of the ii electrons in the higher-free energy antibonding molecular orbital. We would write the hypothetical electron configuration of He_two as [latex](\sigma_{1s})^ii[/latex] [latex](\sigma^*_{1s})^2[/latex] as in Figure 10. The cyberspace energy modify would be cypher, and then there is no driving force for helium atoms to form the diatomic molecule. In fact, helium exists as discrete atoms rather than as diatomic molecules. The bail lodge in a hypothetical dihelium molecule would be zero.

[latex]\text{bond social club in He}_2 = \frac{(2 - 2)}{2} = 0[/latex]

A bail guild of zero indicates that no bond is formed betwixt two atoms.

Figure 10. The molecular orbital energy diagram predicts that He₂ volition not be a stable molecule, since it has equal numbers of bonding and antibonding electrons.

The Diatomic Molecules of the 2d Period

Eight possible homonuclear diatomic molecules might be formed past the atoms of the second menstruum of the periodic table: Li₂, Be₂, B₂, C₂, Northward₂, O_two, F₂, and Ne₂. Yet, we can predict that the Be_two molecule and the Ne₂ molecule would not be stable. We tin see this past a consideration of the molecular electron configurations (Table 3).

Nosotros predict valence molecular orbital electron configurations just as we predict electron configurations of atoms. Valence electrons are assigned to valence molecular orbitals with the lowest possible energies. Consistent with Hund's dominion, whenever there are two or more degenerate molecular orbitals, electrons fill each orbital of that blazon singly before any pairing of electrons takes place.

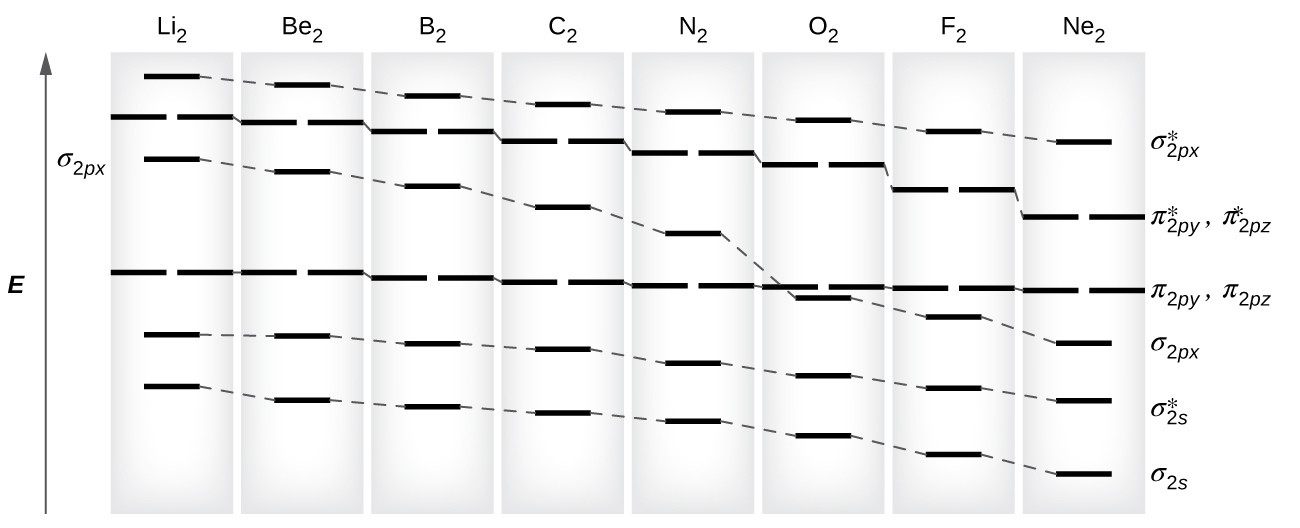
As we saw in valence bail theory, σ bonds are generally more stable than π bonds formed from degenerate atomic orbitals. Similarly, in molecular orbital theory, σ orbitals are usually more stable than π orbitals. Still, this is not always the case. The MOs for the valence orbitals of the second flow are shown in Effigy 11. Looking at Ne_ii molecular orbitals, we see that the lodge is consistent with the generic diagram shown in the previous section. Notwithstanding, for atoms with three or fewer electrons in the p orbitals (Li through North) nosotros discover a different pattern, in which the σ _p orbital is higher in energy than the π _p set. Obtain the molecular orbital diagram for a homonuclear diatomic ion by adding or subtracting electrons from the diagram for the neutral molecule.

Figure xi. This shows the MO diagrams for each homonuclear diatomic molecule in the 2nd period. The orbital energies subtract across the period as the effective nuclear accuse increases and atomic radius decreases. Between N₂ and O_two, the order of the orbitals changes.

You lot tin can practice labeling and filling molecular orbitals with this interactive tutorial from the University of Sydney.

This switch in orbital ordering occurs because of a phenomenon chosen south-p mixing. due south-p mixing does not create new orbitals; it merely influences the energies of the existing molecular orbitals. The σ_s wavefunction mathematically combines with the σ_p wavefunction, with the upshot that the σ_s orbital becomes more than stable, and the σ_p orbital becomes less stable (Effigy 12). Similarly, the antibonding orbitals besides undergo due south-p mixing, with the σ_s* becoming more than stable and the σ_p* becoming less stable.

Figure 12. Without mixing, the MO blueprint occurs every bit expected, with the σ_p orbital lower in free energy than the σ_p orbitals. When s-p mixing occurs, the orbitals shift as shown, with the σ_p orbital higher in energy than the π_p orbitals.

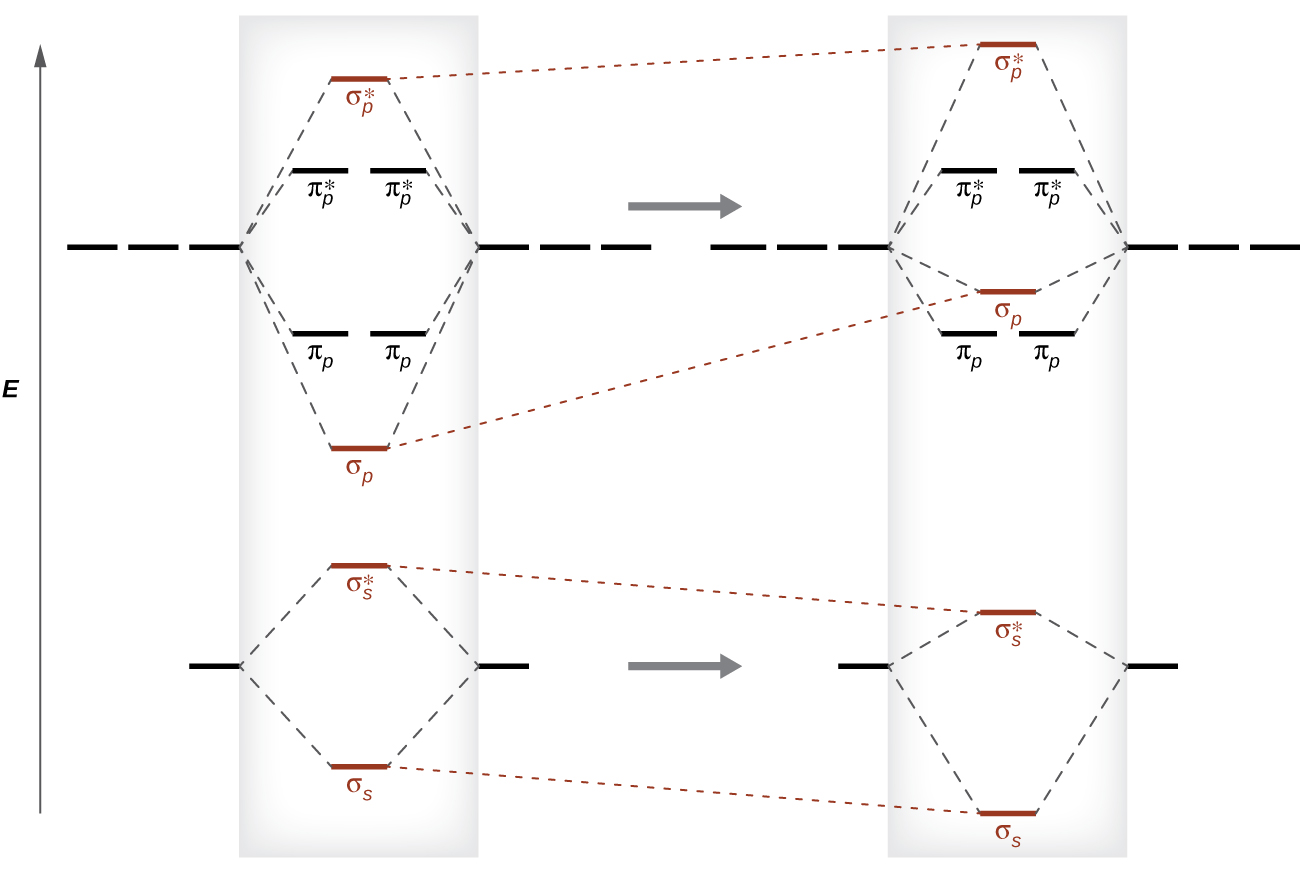
s-p mixing occurs when the s and p orbitals accept similar energies. When a single p orbital contains a pair of electrons, the deed of pairing the electrons raises the energy of the orbital. Thus the twop orbitals for O, F, and Ne are higher in free energy than the 2p orbitals for Li, Be, B, C, and N. Because of this, O_ii, F_two, and North_two simply have negligible due south-p mixing (not sufficient to change the free energy ordering), and their MO diagrams follow the normal pattern, every bit shown in Figure eleven. All of the other period ii diatomic molecules practise take s-p mixing, which leads to the pattern where the σ_p orbital is raised in a higher place the π_p prepare.

Using the MO diagrams shown in Figure xi, we can add in the electrons and determine the molecular electron configuration and bond social club for each of the diatomic molecules. Every bit shown in Table 3, Be₂ and Ne_two molecules would have a bond guild of 0, and these molecules practice non be.

Molecule	Electron Configuration	Bail Society
Litwo	[latex](\sigma_{2s})^two[/latex]	1
Be2 (unstable)	[latex](\sigma_{2s})^2 (\sigma^*_{2s})^2[/latex]	0
B2	[latex](\sigma_{2s})^two (\sigma^*_{2s})^2 (\pi_{2py}, \pi_{2pz})^two[/latex]	1
C2	[latex](\sigma_{2s})^2 (\sigma^*_{2s})^2 (\pi_{2py}, \pi_{2pz})^4[/latex]	two
Due north2	[latex](\sigma_{2s})^2 (\sigma^*_{2s})^2 (\pi_{2py}, \pi_{2pz})^4 (\sigma_{2px})^ii[/latex]	3
O2	[latex](\sigma_{2s})^two (\sigma^*_{2s})^two (\sigma_{2px})^2 (\pi_{2py}, \pi_{2pz})^4 (\pi^*_{2py}, \pi^*_{2pz})^ii[/latex]	ii
F2	[latex](\sigma_{2s})^2 (\sigma^*_{2s})^2 (\sigma_{2px})^2 (\pi_{2py}, \pi_{2pz})^iv (\pi^*_{2py}, \pi^*_{2pz})^4[/latex]	1
Netwo (unstable)	[latex](\sigma_{2s})^two (\sigma^*_{2s})^2 (\sigma_{2px})^2 (\pi_{2py}, \pi_{2pz})^4 (\pi^*_{2py}, \pi^*_{2pz})^4 (\sigma^*_{2px})^2[/latex]	0
Table 3. Electron Configuration and Bond Order for Molecular Orbitals in Homonuclear Diatomic Molecules of Period Two Elements

The combination of two lithium atoms to form a lithium molecule, Li₂, is analogous to the formation of H₂, only the diminutive orbitals involved are the valence twodue south orbitals. Each of the 2 lithium atoms has one valence electron. Hence, we have two valence electrons available for the σ_2s bonding molecular orbital. Because both valence electrons would be in a bonding orbital, nosotros would predict the Li_ii molecule to exist stable. The molecule is, in fact, present in observable concentration in lithium vapor at temperatures near the boiling point of the element. All of the other molecules in Table 3 with a bond order greater than goose egg are besides known.

The O₂ molecule has plenty electrons to one-half fill up the ([latex]\pi^*_{2py}[/latex], [latex]\pi^*_{2pz}[/latex]) level. Nosotros look the two electrons that occupy these two degenerate orbitals to exist unpaired, and this molecular electronic configuration for O₂ is in accord with the fact that the oxygen molecule has two unpaired electrons (Figure 14). The presence of two unpaired electrons has proved to be difficult to explain using Lewis structures, but the molecular orbital theory explains it quite well. In fact, the unpaired electrons of the oxygen molecule provide a strong piece of back up for the molecular orbital theory.

Ring Theory

When ii identical atomic orbitals on different atoms combine, ii molecular orbitals issue (come across Effigy 3). The bonding orbital is lower in energy than the original atomic orbitals considering the diminutive orbitals are in-phase in the molecular orbital. The antibonding orbital is college in free energy than the original diminutive orbitals considering the atomic orbitals are out-of-phase.

In a solid, similar things happen, only on a much larger scale. Remember that even in a modest sample at that place are a huge number of atoms (typically > ten²³ atoms), and therefore a huge number of atomic orbitals that may be combined into molecular orbitals. When Due north valence diminutive orbitals, all of the same free energy and each containing one (1) electron, are combined, Due north/2 (filled) bonding orbitals and North/2 (empty) antibonding orbitals volition result. Each bonding orbital volition show an energy lowering every bit the diminutive orbitals are mostly in-phase, but each of the bonding orbitals volition be a little different and have slightly different energies. The antibonding orbitals volition bear witness an increment in energy as the atomic orbitals are mostly out-of-phase, but each of the antibonding orbitals will also exist a fiddling dissimilar and accept slightly different energies. The allowed energy levels for all the bonding orbitals are so close together that they form a ring, called the valence band. Likewise, all the antibonding orbitals are very close together and form a band, chosen the conduction band. Figure 13 shows the bands for three important classes of materials: insulators, semiconductors, and conductors.

Figure 13. Molecular orbitals in solids are then closely spaced that they are described every bit bands. The valence ring is lower in energy and the conduction band is higher in energy. The type of solid is determined by the size of the "band gap" between the valence and conduction bands. Only a very small amount of energy is required to motion electrons from the valance band to the conduction band in a usher, and then they behave electricity well. In an insulator, the band gap is large, so that very few electrons move, and they are poor conductors of electricity. Semiconductors are in between: they acquit electricity better than insulators, but not besides as conductors.

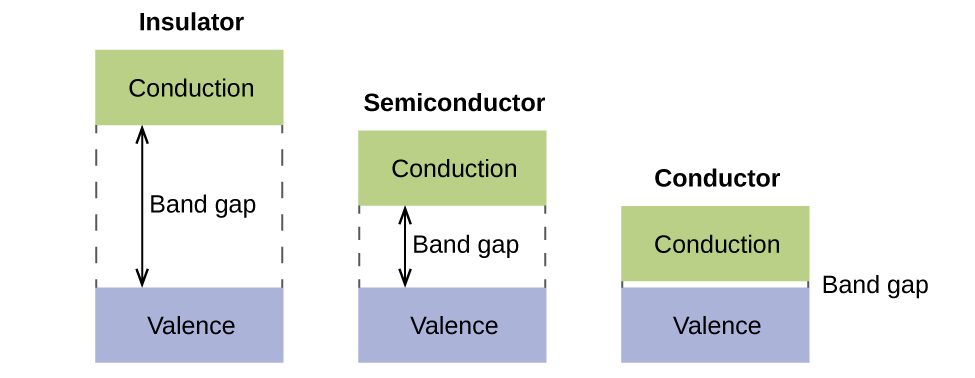
In order to conduct electricity, electrons must move from the filled valence band to the empty conduction ring where they can move throughout the solid. The size of the band gap, or the energy departure betwixt the top of the valence ring and the bottom of the conduction band, determines how easy information technology is to move electrons between the bands. Only a small amount of energy is required in a conductor because the band gap is very small. This small energy deviation is "piece of cake" to overcome, so they are practiced conductors of electricity. In an insulator, the band gap is so "large" that very few electrons move into the conduction band; as a result, insulators are poor conductors of electricity. Semiconductors deport electricity when "moderate" amounts of energy are provided to move electrons out of the valence band and into the conduction band. Semiconductors, such as silicon, are found in many electronics.

Semiconductors are used in devices such every bit computers, smartphones, and solar cells. Solar cells produce electricity when calorie-free provides the energy to movement electrons out of the valence band. The electricity that is generated may then be used to power a calorie-free or tool, or it can be stored for subsequently use by charging a bombardment. Every bit of December 2014, up to 46% of the free energy in sunlight could be converted into electricity using solar cells.

Instance ii

Molecular Orbital Diagrams, Bond Gild, and Number of Unpaired Electrons
Depict the molecular orbital diagram for the oxygen molecule, O₂. From this diagram, calculate the bond society for O₂. How does this diagram account for the paramagnetism of O_ii?

Solution

We draw a molecular orbital free energy diagram similar to that shown in Figure eleven. Each oxygen cantlet contributes six electrons, so the diagram appears as shown in Figure xiv.

Effigy fourteen. The molecular orbital energy diagram for O_two predicts two unpaired electrons.

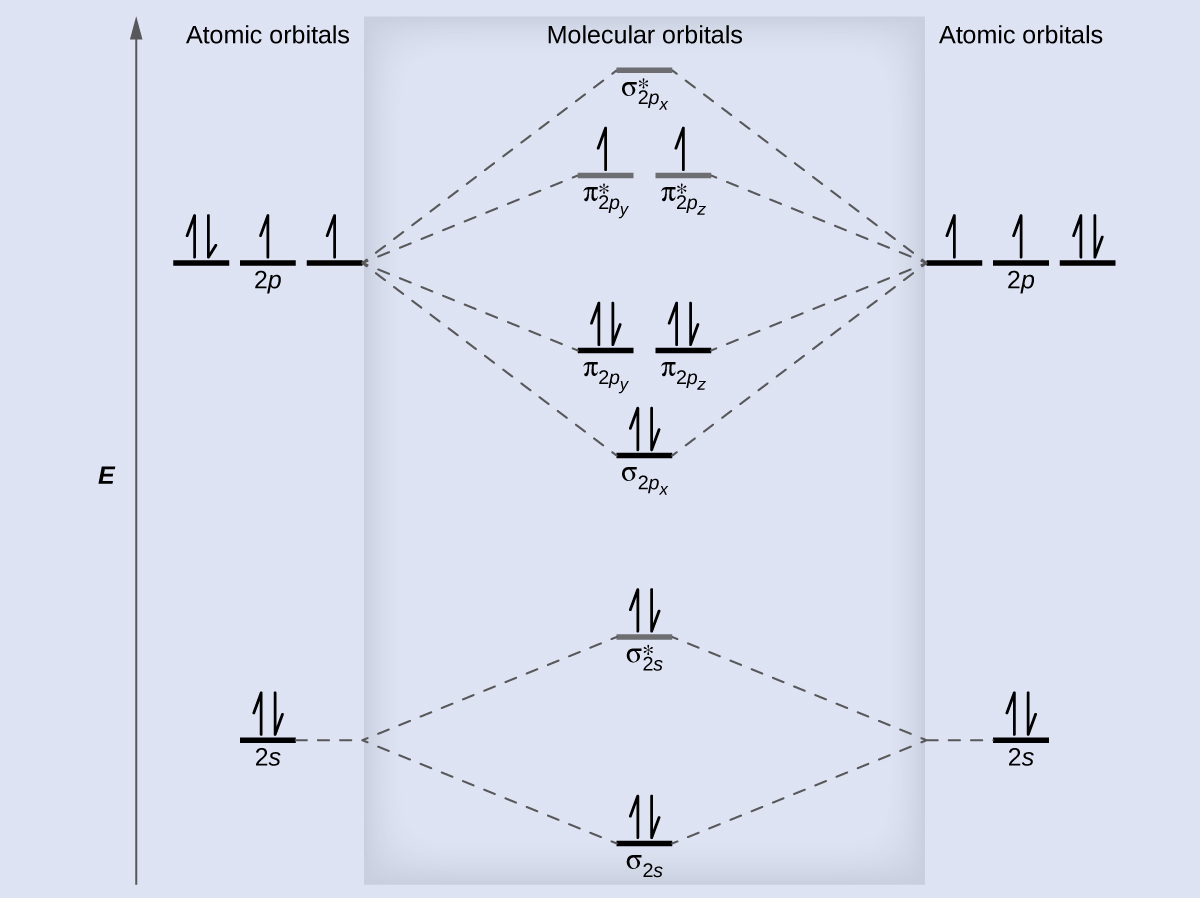
Nosotros summate the bond order equally

[latex]\text{O}_2 = \frac{8 - 4}{2} = 2[/latex]

Oxygen's paramagnetism is explained by the presence of two unpaired electrons in the (π_twopy , π_twopz )* molecular orbitals.

Check Your Learning

The master component of air is Northward₂. From the molecular orbital diagram of N₂, predict its bail social club and whether information technology is diamagnetic or paramagnetic.

Answer:

Due north₂ has a bail gild of 3 and is diamagnetic.

Instance iii

Ion Predictions with MO Diagrams
Give the molecular orbital configuration for the valence electrons in C₂ ^two−. Will this ion exist stable?

Solution

Looking at the appropriate MO diagram, nosotros see that the π orbitals are lower in energy than the σ _p orbital. The valence electron configuration for C₂ is [latex](\sigma_{2s})^2 (\sigma^*_{2s})^2 (\pi_{2py}, \pi_{2pz})^four[/latex]. Adding two more electrons to generate the C₂ ⁱⁱ⁻ anion will give a valence electron configuration of [latex](\sigma_{2s})^2 (\sigma^*_{2s})^2 (\pi_{2py}, \pi_{2pz})^four (\sigma_{2px})^two[/latex]. Since this has vi more bonding electrons than antibonding, the bond gild will exist 3, and the ion should be stable.

Check Your Learning

How many unpaired electrons would exist nowadays on a Be₂ ^two− ion? Would information technology exist paramagnetic or diamagnetic?

Answer:

two, paramagnetic

Creating molecular orbital diagrams for molecules with more than two atoms relies on the aforementioned basic ideas as the diatomic examples presented here. Still, with more atoms, computers are required to calculate how the atomic orbitals combine. See iii-dimensional drawings of the molecular orbitals for C_viH₆.

Key Concepts and Summary

Molecular orbital (MO) theory describes the beliefs of electrons in a molecule in terms of combinations of the diminutive wave functions. The resulting molecular orbitals may extend over all the atoms in the molecule. Bonding molecular orbitals are formed by in-phase combinations of atomic moving ridge functions, and electrons in these orbitals stabilize a molecule. Antibonding molecular orbitals result from out-of-phase combinations of atomic wave functions and electrons in these orbitals make a molecule less stable. Molecular orbitals located forth an internuclear axis are called σ MOs. They can be formed from s orbitals or from p orbitals oriented in an terminate-to-end fashion. Molecular orbitals formed from p orbitals oriented in a side-past-side fashion have electron density on reverse sides of the internuclear axis and are called π orbitals.

We tin can describe the electronic structure of diatomic molecules by applying molecular orbital theory to the valence electrons of the atoms. Electrons fill molecular orbitals following the same rules that apply to filling atomic orbitals; Hund's rule and the Aufbau principle tell the states that lower-free energy orbitals will fill first, electrons will spread out before they pair up, and each orbital tin can hold a maximum of 2 electrons with opposite spins. Materials with unpaired electrons are paramagnetic and attracted to a magnetic field, while those with all-paired electrons are diamagnetic and repelled by a magnetic field. Correctly predicting the magnetic properties of molecules is in advantage of molecular orbital theory over Lewis structures and valence bond theory.

Central Equations

• [latex]\text{bond order} = \frac{(\text{number of bonding electron}) - (\text{number of antibonding electrons})}{2}[/latex]

Chemistry Cease of Chapter Exercises

1. Sketch the distribution of electron density in the bonding and antibonding molecular orbitals formed from two s orbitals and from two p orbitals.
2. How are the following similar, and how do they differ?

   (a) σ molecular orbitals and π molecular orbitals

   (b) ψ for an atomic orbital and ψ for a molecular orbital

   (c) bonding orbitals and antibonding orbitals

3. If molecular orbitals are created by combining five atomic orbitals from atom A and 5 atomic orbitals from atom B combine, how many molecular orbitals will event?
4. Can a molecule with an odd number of electrons ever be diamagnetic? Explain why or why non.
5. Can a molecule with an even number of electrons e'er be paramagnetic? Explain why or why not.
6. Why are bonding molecular orbitals lower in free energy than the parent atomic orbitals?
7. Calculate the bond order for an ion with this configuration:

   [latex](\sigma_{2s})^2 (\sigma^*_{2s})^ii (\sigma_{2px})^two (\pi_{2py} , \pi_{2pz})^four (\pi^*_{2py} , \pi^*_{2pz})^3[/latex]

8. Explain why an electron in the bonding molecular orbital in the H₂ molecule has a lower energy than an electron in the is atomic orbital of either of the separated hydrogen atoms.
9. Predict the valence electron molecular orbital configurations for the post-obit, and state whether they will be stable or unstable ions.

   (a) Na₂ ^two+

   (b) Mg₂ ²⁺

   (c) Al₂ ⁱⁱ⁺

   (d) Si_two ^two+

   (eastward) P₂ ²⁺

   (f) S₂ ²⁺

   (thou) F₂ ⁱⁱ⁺

   (h) Ar₂ ²⁺

10. Determine the bond lodge of each member of the following groups, and make up one's mind which member of each grouping is predicted by the molecular orbital model to accept the strongest bond.

    (a) H_ii, H_ii ⁺, H₂ ⁻

    (b) O₂, O_two ²⁺, O_ii ^two−

    (c) Li₂, Be₂ ⁺, Exist_ii

    (d) F₂, F_two ⁺, F_two ⁻

    (eastward) N₂, N₂ ⁺, Northward₂ ⁻

11. For the first ionization energy for an N₂ molecule, what molecular orbital is the electron removed from?
12. Compare the atomic and molecular orbital diagrams to identify the member of each of the following pairs that has the highest first ionization energy (the nearly tightly bound electron) in the gas phase:

    (a) H and H_two

    (b) North and Northward₂

    (c) O and O₂

    (d) C and C₂

    (e) B and B₂

13. Which of the catamenia two homonuclear diatomic molecules are predicted to be paramagnetic?
14. A friend tells you that the 2s orbital for fluorine starts off at a much lower energy than the 2due south orbital for lithium, so the resulting σ_{2due south} molecular orbital in F_two is more stable than in Li₂. Practice y'all agree?
15. True or imitation: Boron contains iisouthward ²2p ^one valence electrons, then only one p orbital is needed to form molecular orbitals.
16. What charge would be needed on F₂ to generate an ion with a bail society of ii?
17. Predict whether the MO diagram for S_two would show south-p mixing or not.
18. Explicate why Northward₂ ²⁺ is diamagnetic, while O₂ ^four+, which has the same number of valence electrons, is paramagnetic.
19. Using the MO diagrams, predict the bail order for the stronger bond in each pair:

    (a) B₂ or B_ii ⁺

    (b) F₂ or F₂ ⁺

    (c) O_ii or O_two ⁱⁱ⁺

    (d) C_two ⁺ or C_two ⁻

Glossary

antibonding orbital

molecular orbital located outside of the region between two nuclei; electrons in an antibonding orbital destabilize the molecule

bail order

number of pairs of electrons between two atoms; it can be institute by the number of bonds in a Lewis construction or by the difference between the number of bonding and antibonding electrons divided past two

bonding orbital

molecular orbital located between two nuclei; electrons in a bonding orbital stabilize a molecule

degenerate orbitals

orbitals that accept the same energy

diamagnetism

phenomenon in which a material is not magnetic itself only is repelled past a magnetic field; information technology occurs when there are only paired electrons present

homonuclear diatomic molecule

molecule consisting of two identical atoms

linear combination of atomic orbitals

technique for combining atomic orbitals to create molecular orbitals

molecular orbital

region of infinite in which an electron has a high probability of existence found in a molecule

molecular orbital diagram

visual representation of the relative energy levels of molecular orbitals

molecular orbital theory

model that describes the behavior of electrons delocalized throughout a molecule in terms of the combination of atomic wave functions

paramagnetism

miracle in which a material is non magnetic itself simply is attracted to a magnetic field; it occurs when there are unpaired electrons nowadays

π bonding orbital

molecular orbital formed by side-by-side overlap of atomic orbitals, in which the electron density is found on opposite sides of the internuclear centrality

π* bonding orbital

antibonding molecular orbital formed by out of stage side-by-side overlap of atomic orbitals, in which the electron density is found on both sides of the internuclear axis, and there is a node between the nuclei

σ bonding orbital

molecular orbital in which the electron density is institute along the axis of the bail

σ* bonding orbital

antibonding molecular orbital formed by out-of-stage overlap of atomic orbital along the centrality of the bond, generating a node between the nuclei

s-p mixing

change that causes σ _p orbitals to exist less stable than π _p orbitals due to the mixing of s and p-based molecular orbitals of similar energies.

Solutions

Answers to Chemical science Cease of Chapter Exercises

2. (a) Similarities: Both are bonding orbitals that can incorporate a maximum of two electrons. Differences: σ orbitals are stop-to-finish combinations of atomic orbitals, whereas π orbitals are formed by side-past-side overlap of orbitals. (b) Similarities: Both are quantum-mechanical constructs that represent the probability of finding the electron nearly the atom or the molecule. Differences: ψ for an diminutive orbital describes the behavior of merely ane electron at a fourth dimension based on the atom. For a molecule, ψ represents a mathematical combination of atomic orbitals. (c) Similarities: Both are orbitals that can contain two electrons. Differences: Bonding orbitals consequence in holding ii or more atoms together. Antibonding orbitals take the effect of destabilizing any bonding that has occurred.

4. An odd number of electrons tin can never be paired, regardless of the organization of the molecular orbitals. It volition always be paramagnetic.

6. Bonding orbitals have electron density in close proximity to more than i nucleus. The interaction between the bonding positively charged nuclei and negatively charged electrons stabilizes the system.

8. The pairing of the two bonding electrons lowers the energy of the arrangement relative to the free energy of the nonbonded electrons.

x. (a) H₂ bail guild = ane, H_two ⁺ bond lodge = 0.5, H₂ ⁻ bond social club = 0.5, strongest bail is H₂; (b) O₂ bond order = 2, O_two ²⁺ bond lodge = 3; O_two ²⁻ bond social club = 1, strongest bond is O_ii ⁱⁱ⁺; (c) Li_two bond society = 1, Be₂ ⁺ bond order = 0.5, Be_two bond order = 0, strongest bond is Li₂;(d) F_two bond social club = one, F₂ ⁺ bond social club = 1.5, F₂ ⁻ bail order = 0.5, strongest bond is F_ii ⁺; (e) North_two bail order = three, North₂ ⁺ bond order = 2.5, N₂ ⁻ bond order = 2.5, strongest bond is N_two

12. (a) H₂; (b) N₂; (c) O; (d) C_ii; (east) B_two

14. Yes, fluorine is a smaller atom than Li, then atoms in the twos orbital are closer to the nucleus and more stable.

sixteen. 2+

18. N₂ has due south-p mixing, so the π orbitals are the concluding filled in Northward₂ ⁱⁱ⁺. O_two does non take southward-p mixing, and then the σ _p orbital fills before the π orbitals.

Source: https://opentextbc.ca/chemistry/chapter/8-4-molecular-orbital-theory/

Posted by: terrellsuaing.blogspot.com

Share this post